1. Which of the following statements is the definition of parallel lines?
- Two distinct coplanar lines that intersect at a 90°angle.
- Two distinct coplanar lines that do not intersect.
- Two rays with a common endpoint that point in opposite directions.
- Two rays sharing a common endpoint.
2. The definition “a function that takes points in the plane as inputs and gives other points as outputs” refers to which of the following terms?
- Coincident
- Invariant
- Mensuration
- Transformation
3. If trapezoid JKLM, shown below, was rotated 180° clockwise about the origin, determine which notation would represent the new coordinates of J’ K’ L’M’?

- (x,y)→(-x,-y)
- (x,y)→(y,-x)
- (x,y)→(-y,x)
- (x,y)→(y,x)
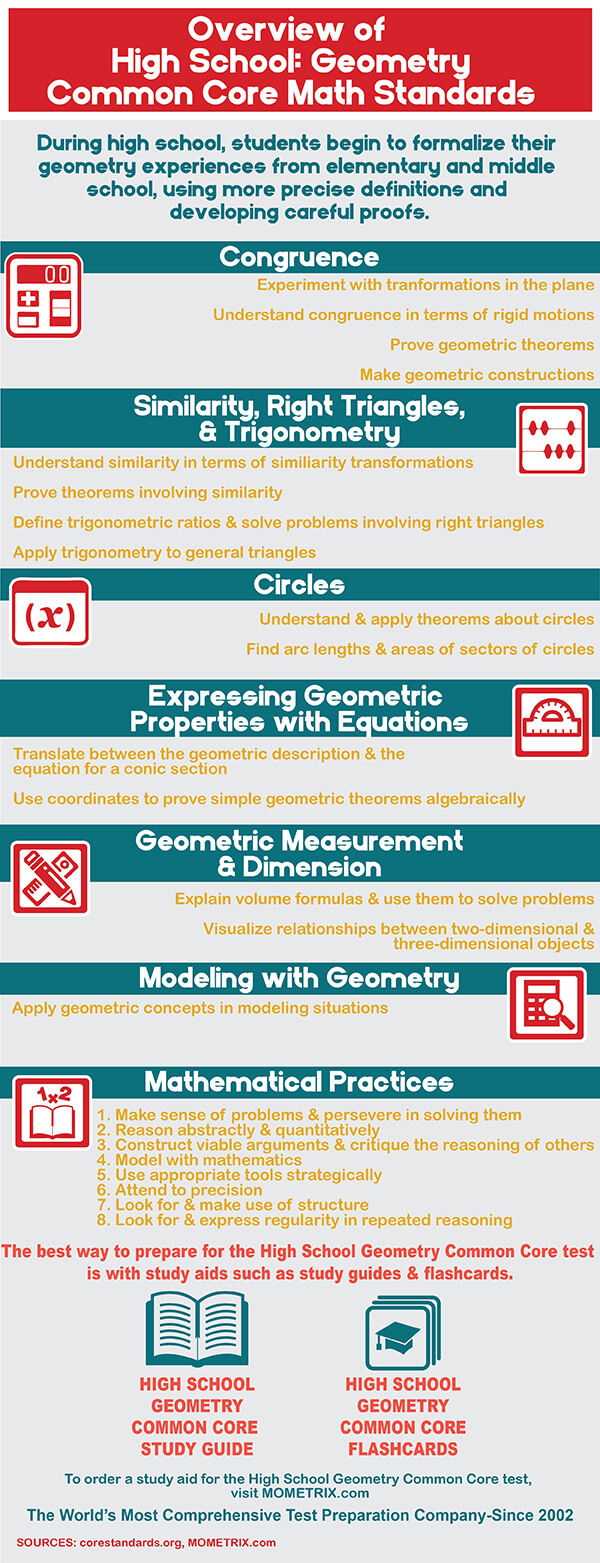
4. Which of the following figures show parallelogram WXYZ being carried onto its image W’X’Y’Z’ by a reflection across the x-axis?
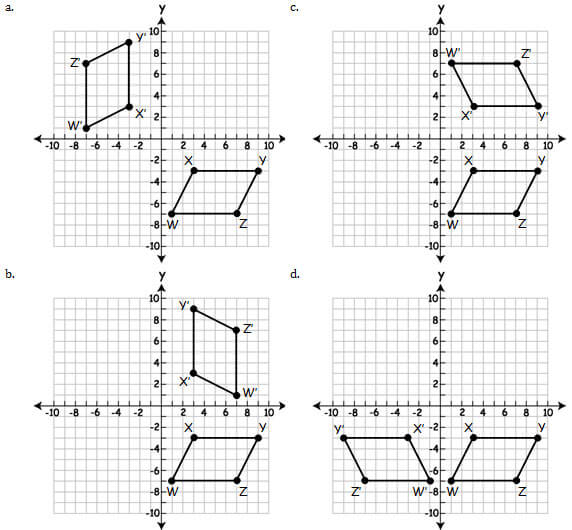
5. If ‾STis reflected across the line y = x, what is the new coordinate point of T’?
- (7,4)
- (-7,- 4)
- (4,-7)
- (-4,7)
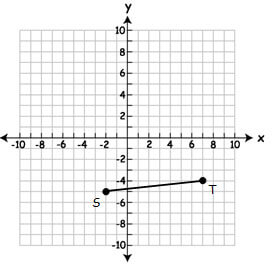
6. Which of the following figures has been rotated 90° clockwise about the origin?
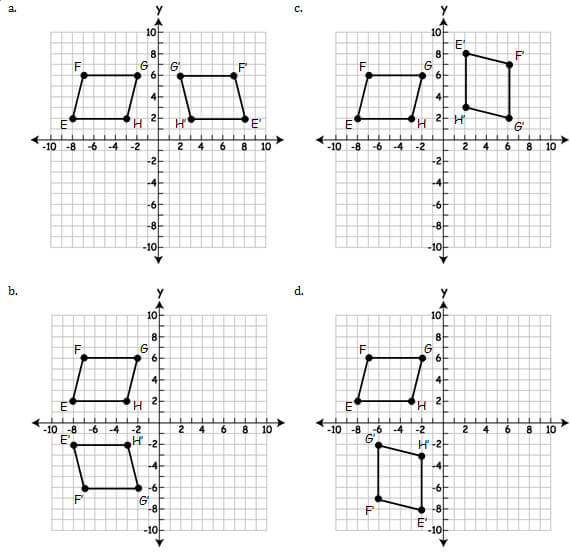
7. Which of the following rules describes the translation of JKLM to its image J’K’L’M’?
- (x,y)→(x-6,y+8)
- (x,y)→(x+6,y-8)
- (x,y)→(x-8,y+6)
- (x,y)→(x+8,y-6)
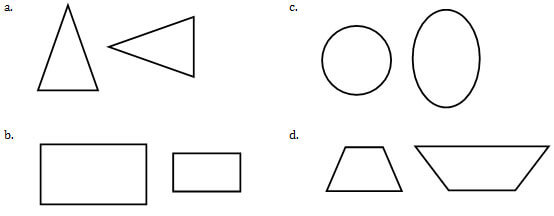
8. Which set of figures is congruent?
9. Which of the following is true about the relationship between the two triangles shown below?
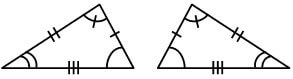
- The triangles are similar.
- The triangles are congruent.
- The triangles are equilateral.
- Both Answer A and Answer B are true.
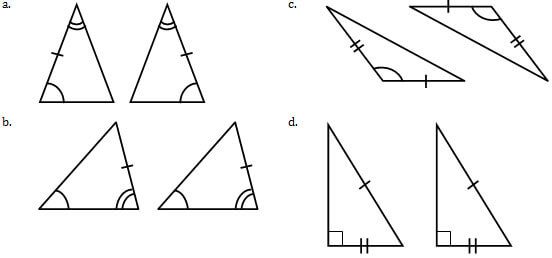
10. ASA triangle congruence can be used to prove which of the following pairs of triangles congruent?
Answers and Explanations
1. B: Parallel lines are two distinct lines in the same plane that do not intersect. Answer A is the definition of perpendicular lines. Answer C is the definition of opposite rays, which form a line. Answer D is the definition of an angle.
2. D: A transformation is described as a function that takes points in the plane as inputs and gives other points as outputs. In Answer A, coincident means that two images are superimposed on one another. In Answer B, invariant means a property that cannot be changed by a given transformation. In Answer C, mensuration is the measurement of geometric figures, such as length, area, angle measure and volume.
3. A: A 180° clockwise rotation about the origin takes the original coordinates and negates them. Therefore, the original coordinates of (x,y) become (-x,-y) after the rotation. Answer B is the coordinate change after a 90° clockwise rotation about the origin. Answer C is the coordinate change after a 90° counterclockwise rotation about the origin. Answer D is the Answer B is the coordinate change after a reflection across the line y = x.
4. C: A reflection is a transformation producing a mirror image. A figure reflected over the x-axis will have its vertices in the form (x,y) transformed to (x,-y). The point W at (1,-7) reflects to W’ at (1,7). Only Answer C shows WXYZ being carried onto its image W’X’Y’Z’ by a reflection across the x-axis. Answer A shows a reflection across the line y = x. Answer B shows a 90° counterclockwise rotation about the origin. Answer D shows a reflection across the y-axis.
5. D: For a reflection across the line y = x, the original coordinate points of (x,y) reverse to become (y,x) for the image. Therefore, since T is located at (7,-4), the coordinates of T’ after the reflection across the line y = x become (-4,7). Answer A is for a reflection across the x-axis. Answer B is for a reflection across the y-axis. Answer C is for a 90° clockwise rotation about the origin.
6. C: Since EFGHis initially located in Quadrant II, a 90° clockwise rotation will rotate the image into Quadrant I. As EFGH is rotated 90° clockwise, each vertex of the figure will undergo the coordinate transition of (x,y) →(y,-x), as is the case in Answer C. Answer A is a reflection across the y-axis. Answer B is a reflection across the x-axis. Answer D is a 90° counterclockwise rotation.
7. B: To determine the translation, compare the coordinates of J(-4,1) and J'(2,-7). To find the change in the x-direction, subtract the x coordinate of the starting position from the final position that is Δx=2-(-4)=2+4=6. Similarly, the change in the y-direction is -7-1=-8. We can check that the same is true for the other vertices. Therefore, the rule that describes the translation of JKLM to its image J’K’L’M’ is (x,y)→ (x+6,y-8). Answer A incorrectly used the opposite signs. Answer C incorrectly used the opposite signs and had the x and y directions reversed. Answer D had the x and y directions reversed.
8. A: In order for two figures to be congruent, they must have the same size and shape. The two triangles in Answer A have the same size and shape, even though the second triangle is rotated counterclockwise compared to the first triangle. The rectangles in Answer B are the same shape, but they are not the same size. In Answer C, the second circle has been vertically stretched, so the figures are not the same shape or size. In Answer D, the second trapezoid has been horizontally stretched so it is not the same size as the first trapezoid.
9. D: Since the two triangles have all three corresponding pairs of sides and corresponding pairs of angles marked congruent, then the two triangles are congruent. Similar triangles are the same shape but not necessarily the same size; they have congruent angles. All congruent triangles are similar triangles, so Answer D is the best choice. In Answer C, equilateral triangles are triangles that have sides with all the same measure within the same triangle, not in relation to another triangle.
10. A: In order for triangles to be congruent by ASA, there needs to be two pairs of congruent corresponding angles and then the pair of sides between those angles is also congruent. The triangles in Answer A meet the requirements for ASA triangle congruence. In Answer B, the triangles are congruent by AAS. In Answer C, the triangles are congruent by SAS. In Answer D, the triangles are congruent by HL (Hypotenuse Leg).